Sharpe Ratio

What is Sharpe Ratio?

Sharpe Ratio measures returns with respect to the risk taken. The returns can be both negative and positive. A higher Sharpe ratio means, a higher return without too much risk. Thus, while Investing, investors should choose a fund that shows a higher Sharpe ratio. Sharpe Ratio comes very handy to measure the risk-adjusted returns potential of a Mutual Fund.

The Sharpe ratio named after Stanford professor and Nobel laureate William F. Sharpe.

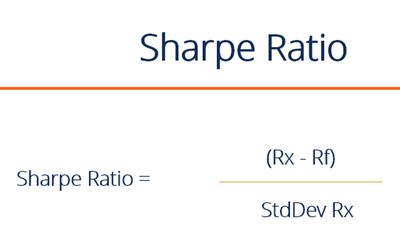
Formula for Sharpe Ratio

We will give you a better understanding of how this ratio works, starting with its formula:

S (x) = (rx - Rf) / StdDev (x)

Where:

X is the investment rx is the average rate of return of X Rf is the best available rate of return of a risk-free security (i.e. T-bills) StdDev(x) is the Standard Deviation of rx

Sharpe Ratio Calculation

Using the Sharpe ratio formula, let's assume for illustration purpose that you expect your stock Portfolio to return 15 percent next year. If returns on risk-free Treasury notes are, say, 7 percent, and your portfolio carries a 0.06 standard deviation, then from the formula we can calculate that the Sharpe ratio for your portfolio is:

(0.15 - 0.07)/0.06 = 1.33

This means that for every point of return, you are shouldering 1.33 units of risk.

Sharpe Ratio Grading Thresholds

• <1: Not Good
• 1 – 1.99: Ok
• 2 – 2.99: Really Good
• *>3: Exceptional

Portfolios with higher rates of risk might have a metric of 1, 2, or 3. Any metric equal to or greater than 3 is considered a great Sharpe measurement and a good investment all else equal.