Table of Contents
Defining Effective Interest Method
The effective interest method is a bond amortisation approach that shows the Real Interest Rate in effect at any point in the bond's life before maturity. It is calculated using the bond's Book Value at the start of each Accounting period. When buying Bonds, there are two separate interest rates to consider:
- the stated rate, and
- the Market rate

The stated rate is the one that is written on the bond. The market rate is the interest rate at which the bond market is willing to pay at the time. Both of them are rarely the same since bonds might take many months to be issued to investors once they are drafted.
When an investor buys a bond at a premium or a discount to its Face Value, this calculation can be quite useful. Bond premium means when investors are ready to pay more than the face value of a bond because its stated interest rate is greater than the prevailing market interest rate. When investors are only prepared to pay less than the face value of a bond because its stated interest rate is lower than the actual market rate, this is known as a bond discount.
Formula to Calculate Effective Interest Rate
The effective interest rate, which is a major component of the calculation, discounts the projected future cash inflows and outflows during the life of a Financial Instrument when using the effective interest approach.
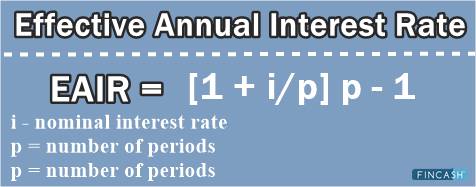
In general terms, the effective interest rate multiplied by the carrying value of a financial instrument equals the interest Income or cost reported in a reporting period. Let’s learn how effective interest rate is calculated mathematically. So, here is the formula used for the same.
Effective Interest Rate = [ (1+ I/N)^N-1 ] * Current book value
Where,
- I = Rate of bond’s coupon
- N = number of coupon payments per year
Talk to our investment specialist
Why prefer Effective Interest Method over Straight Line Method?
The effective interest approach is far more accurate than the straight-line approach. It is, however, more complicated to calculate than the straight-line approach since the effective method must be recalculated every month, whereas the straight-line method charges the same amount each month. It's usually only used when a bond is bought at a substantial discount or premium or when the bond's book value rises or falls dramatically throughout the bond's life.
The straight-line amortisation technique works fine and is easier to compute for a bond acquired at face value with a generally consistent book value over the bond's life to maturity. In any case, the straight-line amortisation technique and the effective interest rate method of calculating amortisation will be the same when the bond reaches maturity.
Application Of Effective Interest Method
Let’s look at the application of effective interest method calculation areas:
- When bonds or Debentures are issued at premium or discount.
- While calculating the current value of minimum Lease payments.
- While calculating the current value of security deposits under International Financial Reporting Standards (IFRS).
Benefits of Effective Interest Method
- There is no unexpected expense or profit in the profit and loss statement. The bond's discounts and premiums are spread out over the bond's life.
- The future impact on the profit and loss account is understood in advance, allowing for a more precise interest budgeting.
- This method employs more advanced accounting techniques, such as the matching idea.
All efforts have been made to ensure the information provided here is accurate. However, no guarantees are made regarding correctness of data. Please verify with scheme information document before making any investment.